When I was a kid I used to ride the Cyclone at Coney Island. It was an exhilarating experience, but it was always over too soon. So many things in life are like that.
It occurred to me today that if roller coasters were designed by mathematicians, such limitations could be removed. Through the application of some simple principles of geometric topology, I now present, for your consideration, a sketch of a roller coaster mathematically guaranteed to provide an infinite ride.
The fun never has to end. 🙂
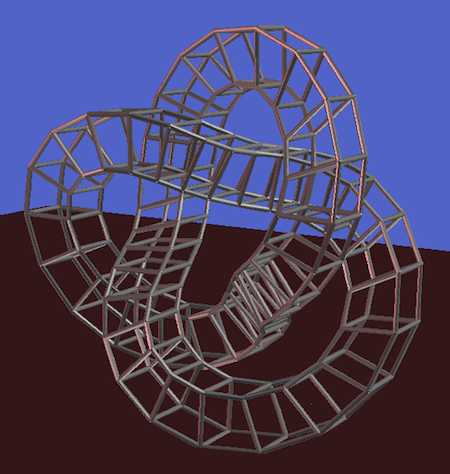
I’m surprised you didn’t twist it too, like a mobius strip.
OMG Sharon, would you really want to be stuck forever on a ride that would make your lunch come back up?
A Möbius strip is a wondrous thing, but it’s a little too twisted for an unending roller coaster ride.
Although maybe I’m being a little too one-sided on the issue. 😉
Personally, even the finite and quick roller coasters are too much for me. But I figured you roller coaster afficianados had iron stomachs already. Do you really think the extra twist would make such a difference? That figure in your picture is already pretty twisty!
You’re probably right. The best thing is just to roll with it. No sense getting tied in knots!
I now see that this entire blog post was just an excuse for puns. Not that there is anything wrong with that 🙂
You know, it is probably better that the thrill of a roller coaster ride is short-lived. If it went on too long the thrill would wear off and the riders would start looking for the next exciting thing. So many things in life are like that too.