I would like to use my 3D printer to print the sort of patch-work sphere I talked about in yesterday’s post. The first step in doing that is to model the piece in computer software. Below are two views — a front view followed by a more edge-on view, of the software model:
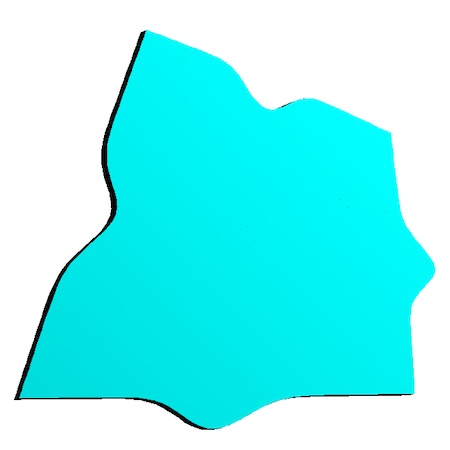
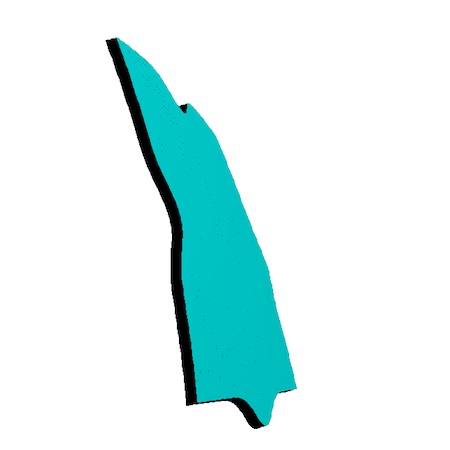
It occurs to me that this would be a great kind of jigsaw puzzle — especially if I modify the edges to make them more interlocking. I did a bit of searching on-line last night to see whether anyone has taken this approach before to designing jigsaw puzzles on spheres, but all the spherical jigsaw puzzles I’ve found are build from a latitude/longitude grid — so the pieces get smaller toward the two poles. Of course the approach I’m describing might already be out there, and it’s just that I couldn’t find it.
If we’re making a jigsaw puzzle, the pieces could form some cool spherical image — the surface of a planet like Earth or Jupiter comes to mind — to distinguish the pieces from each other. Since every shape would be identical, the only way to solve such a puzzle would be through that image.
As M.C. Escher pointed out (as illustrated below in his wonderful 1959 work “Flying Horses”), you can change the edges of this sort of tiling — the only difference here is that we are doing this on a filing that covers a sphere:

It would really be fun to design a puzzle shape that matches the theme (eg: “planet earth” or “moon” or “jack-o-lantern”) of the jigsaw puzzle.
I found some by searching for “3d puzzle spheres” and “3d spherical puzzles”. for example: http://www.jigsawjungle.com/code/ravens/xbpink.htm
oh, here’s the earth: http://www.jigsawjungle.com/code/unicorn/pmearth.htm
Cool, thanks! Now I don’t have to go through all the trouble of actually building one. 🙂
Can you find anyone out there doing a spherical version of Escher’s variable tilings?
I’m surprised, Ken! Since when would you let the pre-existence of something stop you from building it yourself if you were curious? 😉
Or how about an animated globe?
The modularity of Escher’s patterns and the transformations of Huff’s parquetts are begging to be brought into the digital realm – whether 2D or 3D…
http://www.cgl.uwaterloo.ca/~csk/projects/morph/